H. Berg,"Random Walks in Biology", Ch. 1,2 (Princeton University Press, 1983)
R. Feynman, "Lectures on Physics", Vol. 1, Ch.43
J. Crank, "The Mathematics of Diffusion" (Oxford University Press, 1956; 2nd ed. 1976)
Parameters:Diffusion coefficients for
CO2 in air: 0.16 cm2/sec
O2 in air: 0.2 "
H2O in air: 0.25 "
PO4 in water: ~1 x 10-5 cm2/sec
ATP in cytoplasm: 0.15 x 10-5 cm2/sec
O2 in water: 2 x 10-5 cm2/sec
CO2 in water: 1.6 x 10-5 cm2/sec
O2 in air: 9.4 x 10-3 M
PO4 in sea water: 1 to 3 x 10-6 M
O2 in sea water in equilibrium with atmosphere: 2.5 to 3 x 10-4 M
D is a diffusion coefficient, which we usually assume to be a constant.
One dimensional diffusion occurs when we have two infinite parallel boundary planes and concentrations that are constant along any plane parallel to these boundaries. It can also be used exactly for diffusion in a straight tube of constant diameter, and it is often a useful approximation to real situations that do not exactly meet these requirements.Many situations in which we are interested are steady-state situations, in which dC/dt = 0. In simple cases, steady-state solutions for the diffusion equation are usually easier to find than complete, time-dependent solutions. However, in more complex situations, the easiest way to find the steady-state solution may be by numerical integration (with a computer) of the complete equation until an equilibrium is nearly reached.
In aquatic ecosystems, photosynthesis occurs in the euphotic zone , the upper layers in which light intensity is sufficent for photosynthesis to exceed respiration. This may be up to 100 meters in depth. In this region, photosynthesis is accompanied by a net synthesis of organic materials, which must involve the incorporation of nutrients, such as phosphorus which is needed for nucleic acids and other biomolecules. Because this organic material is concentrated in organisms that are heavier than water, eventually it will fall out of the euphotic zone. Some process is needed to return P to the euphotic zone, in order for life in this zone to continue. After the organic material falls below the euphotic zone, it will eventually be oxidized, and the phosphorus will enter the sea water in the form of phosphate ion. However, the concentration of phosphate that can be attained is limited by the solubility product of calcium phosphate minerals, and will not get much above 3 x 10-6 M. Could simple diffusion of phosphate ion from deeper waters towards the surface contribute significantly to the needed recirculation of phosphorus?
We can approach this as a one-dimensional steady-state problem. Therefore, the concentration gradient must be constant over the range we are considering. We assume that the concentration at some depth, say 1000 meters, is maintained at 3 M, and that the concentration falls to 0 at the surface.
We can compare this with an estimate of oceanic net productivity of approximately 70 gm of carbon per year per square meter.Obviously, diffusion does not solve this problem. But wait! If the productivity of 70 gm C per year per m2 is made possible by recycling of phosphate in the euphotic zone, and only a tiny fraction is actually lost by gravitational settling, there might be no problem.
Again, diffusion is inadequate, by a factor of 3 x 10-4. However, in this case, the problem is resolved by a mechanism that uses similar mathematics. Because of the heating of the surface by solar radiation, air near the surface will get hot and rise. This leads to a large amount of convective turbulence, and this turbulence increases the effective diffusion coefficient by a factor of 4 to 5 orders of magnitude. This leads to a result that is consistent with experimental measurements that a cornfield can grow at high rates, that there is only a slight (perhaps 10%) decrease in carbon dioxide concentration near the surface, and that in a few cases such as this it is possible to increase crop yield by supplying exogenous carbon dioxide.
Example: uptake of nutrient molecules by a spherical cell
This is a steady-state problem involving spherical symmetry. The appropriate equation could be derived from the general vector equation, but let's instead use a simpler approach. In the steady state, we assume that all of the molecules reaching the surface of the cell at radius a are adsorbed, resulting in C=0 at r=a, and a constant rate, Q, of uptake of nutrient molecules. This means that the total flux through any spherical shell surrounding the cell must also equal Q. Therefore, for any radius, r;Q = -4pr2 D dC/dr = constant, or dC/dr = -Q/4p r2D.
ThereforeC = Co - Q/4pDr, where Co must be the concentration at r=infinity.
Since C=0 at r=a, we find that Q = 4pDaCo.
Let's compare the effects on two hypothetical cells:
P content:: 8 x 10-14 moles/cell _or_ 8 x 10-11 moles/cell (assume Co=1 M=10-9 moles/cm3 and D=10-5 cm2/sec)
P uptake:: 1.3 x 10-16 moles/sec _or_ 1.3 x 10-15 moles/sec
Doubling time:: 500 sec=~8 min _or_ 50,000 sec = ~14 hours
Can a cell increase its uptake of a nutrient by stirring the surrounding medium with flagella, or by swimming rapidly through the medium so that it is always exposed to a high concentration?A simple-minded (and incorrect) approach: Assume that a spherical cell swims through the medium at velocity V, and absorbs all of the molecules in a cylinder with a radius equal to the radius of the cell -- e.g. pr2 x the distance travelled. The uptake will then be pr2CoV. We can calculate that the velocity required for a small cell (10 microns radius) to double its phosphate uptake over that supplied by diffusion is 400 microns/sec, which is much faster than observed swimming velocities for such cells.
For the large cell (100 microns radius) the velocity is 40 microns/sec, which is reasonable. However, this "barn door" approach is wrong, because it neglects the fact that a small object moving through a fluid will drag fluid along that is sticking to its surface.The correct treatment is given in a classic paper by Berg & Purcell (Biophysical Journal 20: 193-219, 1977). The true relationship between uptake and swimming speed is non-linear. The uptake can be doubled by a swimming speed equal to about 2.5 D/a. (250 microns/sec for the small cell or 25 microns/sec for the large cell) However, to triple the uptake requires a swimming speed of about 20 D/a. (2000 microns/sec for the small cell or 200 microns/sec for the largecell). This method for increasing uptake is worthwhile for a dinoflagellate that can swim at 200 microns/sec, barely useful for a cell the size of Chlamydomonas that can swim at 100 microns/sec, and useless for a bacterium that might be able to swim at 20 microns/sec. It does mean that the disadvantage of being large is not quite so great as indicated by our initial calculation, if the large cell can swim at a reasonable velocity. Swimming efficiency may not be important, if the limiting factor is nutrient availability rather than energy input.
Diffusion of a molecule that is also being consumed by a chemical reaction.In a simple one-dimensional situation, dC/dt = Dd2C/dx2 -q, where q is the rate of the chemical reaction.This can be used, for instance, for the diffusion of ATP along the length of a flagellum, if we assume that [ATP] is everywhere high enough that q is independent of C. The steady state solution is easily found to be:
C = Co -(q/D)(xS-x2/2), where Co is the concentration at x=0, and S is the length of the flagellum. The concentration at x=S is C(S) = Co -(q/D)S2/2, and the solution is only valid if C(S)>>0. Also, if C(S)-->0, we have a situation where the rate of diffusion is not adequate to supply the chemical reaction. We can define a dimensionless criterion:
Ø = qS2/DC where C is the concentration at the input boundary.
If Ø <<>
An example: flight muscles of insects and birds are probably the most actively metabolizing tissues known. A value given for the honeybee is 2.2 kcal/gm of muscle/hour (about 2.5 watts/gm). This metabolism will require 0.37 liters of O2 per hour, or 4.4 x 10-6 moles per cm3 per second. For Ø=1, and assuming that the outer boundary of a mass of tissue is exposed to atmospheric concentrations of oxygen,
S = 0.8 x 10-3 cm = 8 microns.
This gives us a rough estimate of the size of a cell or a mass of tissue, exposed to atmospheric oxygen concentrations, that can easily maintain this high metabolic rate.
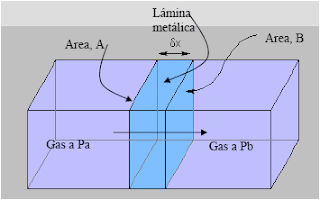
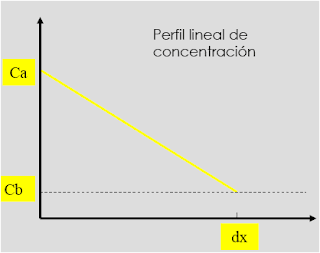




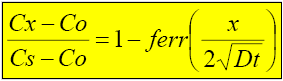 Donde:
Donde:















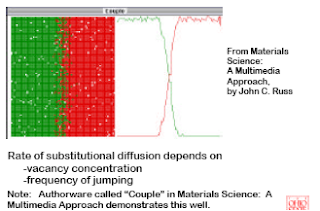
 PROCESSING USING DIFFUSION
PROCESSING USING DIFFUSION


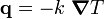
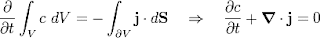 Combinando el resultado anterior con la ley de Fick resulta la ecuación de difusión o segunda ley de Fick:
Combinando el resultado anterior con la ley de Fick resulta la ecuación de difusión o segunda ley de Fick:


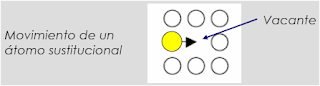
 En la figura de arriba se muestran 6 átomos ordenados en una estructura cristalina hipotética en un material. Cada átomo ocupa un punto de red definido por la celda unitaria correspondiente. Los átomos están unidos por enlaces químicos, y la distancia entre los átomos es la necesaria para que la energía total de los mismos sea la menor posible. Las posiciones de equilibrio de estos átomos pueden ilustrarse de la siguiente manera:
En la figura de arriba se muestran 6 átomos ordenados en una estructura cristalina hipotética en un material. Cada átomo ocupa un punto de red definido por la celda unitaria correspondiente. Los átomos están unidos por enlaces químicos, y la distancia entre los átomos es la necesaria para que la energía total de los mismos sea la menor posible. Las posiciones de equilibrio de estos átomos pueden ilustrarse de la siguiente manera:

 donde:
donde: Fick modeló el flujo de átomos en un material por medio de la siguiente ecuación:
Fick modeló el flujo de átomos en un material por medio de la siguiente ecuación:










